About Progressive Damage and Failure

Abaqus offers a general framework for material failure modeling that allows the combination of multiple failure mechanisms acting simultaneously on the same material. Material failure refers to the complete loss of load-carrying capacity that results from progressive degradation of the material stiffness. The stiffness degradation process is modeled using damage mechanics.

To help understand the failure modeling capabilities in Abaqus, consider the response of a typical metal specimen during a uniaxial tensile test. Figure 1(a), shows the nominal (or engineering) stress-strain response measured directly in a tensile test. The nominal stress reaches a maximum (ultimate tensile strength) value at point $x$ , corresponding to the onset of incipient necking. Beyond this point, deformation localizes in a neck region and the stress-strain state is not uniform in the specimen. Therefore, after point $x$ , the curve is no longer representative of the actual stress-strain state at any given location of the specimen. At point $c$ , there is a marked reduction of load-carrying capacity until rupture occurs, $c-d$ . Due to the nonhomogeneous deformation in the specimen, the nominal stress-strain curve cannot be used directly to characterize the material model. Instead, Abaqus requires the material model to be defined in terms of the true stress-strain response.

Figure 1(b) shows the true stress-strain response at the critical location of the specimen where failure occurs. The labels $a$ , $b$ , $x$ , $c$ , and $d$ on this curve indicate the local true stress-strain states of the material corresponding to each stage of the nominal stress-strain curve (Figure 1(a)). The material response is initially linear elastic, $a-b$ , followed by plastic yielding with strain hardening, $b-c$ . The ultimate strength point, $x$ , lies on the strain-hardening portion of the true stress-strain curve. Beyond point $c$ , there is a marked reduction of load-carrying capacity until rupture, $c-d$ . Point $c$ in this curve identifies the material state at the onset of damage, which is referred to as the damage initiation criterion. Beyond this point, the true stress-strain response $c-d$ is governed by the evolution of the degradation of the stiffness leading to complete failure. In the context of damage mechanics, $c-d$ can be viewed as the degraded response of the curve $c-d^{\prime }$ that the material would have followed in the absence of damage.

Figure 1. Typical uniaxial stress-strain response of a metal specimen: (a) nominal stress-strain response and (b) true-stress strain response at the critical location of failure.

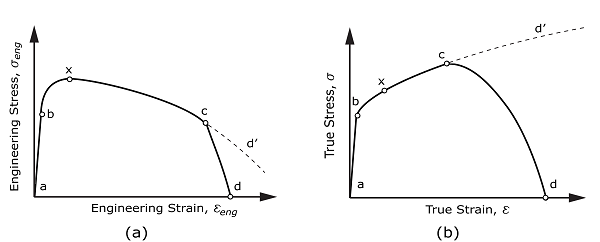
Thus, in Abaqus, you must specify a failure mechanism in terms of the true stress-strain response, consisting of four distinct parts:

• The definition of the effective (or undamaged) material response (for example, $a-b-c-d^{\prime }$ in Figure 1(b))

• A damage initiation criterion (for example, $c$ in Figure 1(b))

• A damage evolution law (for example, $c-d$ in Figure 1(b))

• A choice of element deletion where elements can be removed from the calculations once the material stiffness is fully degraded (for example, $d$ in Figure 1(b))

These parts are discussed separately for ductile metals (About Damage and Failure for Ductile Metals) and fiber-reinforced materials (About Damage and Failure for Fiber-Reinforced Composites).

In continuum mechanics the constitutive model is normally expressed in terms of stress-strain relations. When the material exhibits strain-softening behavior, leading to strain localization, this formulation results in a strong mesh dependency of the finite element results in that the energy dissipated decreases on mesh refinement. In Abaqus all of the available damage evolution models use a formulation intended to alleviate the mesh dependency. This is accomplished by introducing a characteristic length into the formulation, which in Abaqus is related to the element size, and expressing the softening part of the constitutive law as a stress-displacement relation. In this case the energy dissipated during the damage process is specified per unit area, not per unit volume. This energy is treated as an additional material parameter, and it is used to compute the displacement at which full material damage occurs. This is consistent with the concept of critical energy release rate as a material parameter for fracture mechanics. This formulation ensures that the correct amount of energy is dissipated and greatly alleviates the mesh dependency.