Demand wettability of a porous medium: coupled analysis

The properties pertaining to the partially saturated flow behavior of the material are the same as those used in Partially saturated flow in a porous medium. For the mechanical properties we assume the material is elastic, with Young's modulus 10000 Pa and Poisson's ratio 0.0. The mechanical properties of the gel particles are assumed to be similar to those of a fluid since they are mostly made up of absorbed fluid. Therefore, a bulk modulus of 2.0 × 10⁹ Pa is specified for the gel.

The initial conditions for pore pressure and saturation are assumed to be those at the beginning of the absorption curve, so the initial saturation is 0.05 and the initial pore pressure is −10000 Pa.

In the first step of the analysis we establish stress equilibrium in the original configuration of the column of material. A stress of 500 Pa is applied to the mesh to balance the initial pore pressure and saturation conditions. The effective stress principle $\overline{\mathit{\sigma }}=\mathit{\sigma }+su\mathbf{I}$ (s is the saturation and u is the pore pressure) then gives zero effective stresses, $\overline{\mathit{\sigma }}$, for the undeformed configuration.

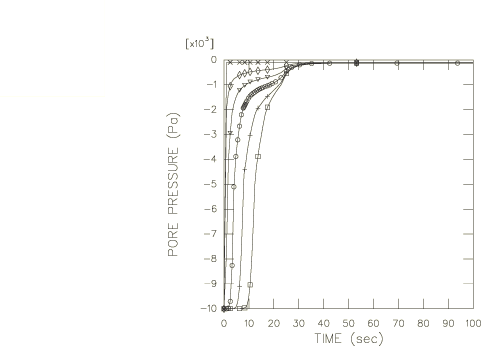
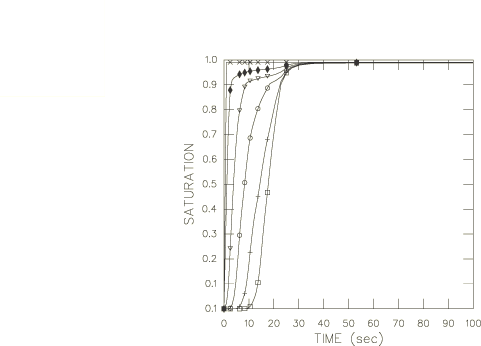
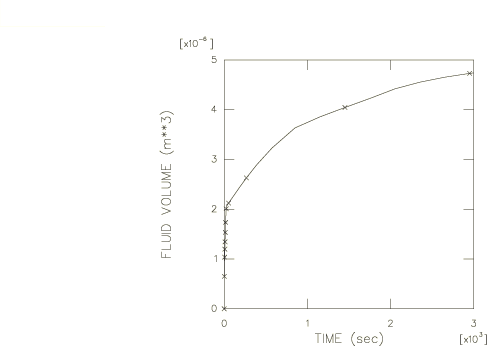
The “loading” consists of prescribing essentially zero pore pressure (corresponding to full saturation) at the bottom of the column. This is based on the assumption that, in the demand wettability test, the sample has available to it as much fluid as necessary to cause saturation at that point. This boundary condition is held fixed for 3000 seconds to model the fluid acquisition process.

The analysis is performed with the transient soils consolidation procedure using automatic time incrementation. The pore pressure tolerance that controls the automatic incrementation is set to a large value since we expect the nonlinearity of the material to restrict the size of the time increments during the transient stages of the analysis and we do not wish to impose any further control on the accuracy of the time integration.

The choice of initial time increment in these transient partially saturated flow problems is important for some element types, to avoid spurious solution oscillations. This is discussed in Partially saturated flow in a porous medium. As discussed in Coupled Pore Fluid Diffusion and Stress Analysis, the criterion for a minimum usable time increment in partial-saturation conditions is

where $\gamma$ is the specific weight of the wetting liquid, $n^0$ is the initial porosity of the material, k is the fully saturated permeability of the material, $k_s$ is the permeability-saturation relationship, $ds/du$ is the rate of change of saturation with respect to pore pressure as defined in the absorption/exsorption material behavior (Sorption), and $\mathrm{\Delta }\mathrm{\ell }$ is a typical element dimension. For our model we have $\mathrm{\Delta }\mathrm{\ell }=$ 5.08 mm (the size of an element side), $\gamma =$ 1.0 × 10⁴ N/m³, $k=$ 3.7 × 10⁻⁴ m/sec, $k_s=s^3$, and $n^0=$ 5/6. Adjacent to where we apply the fully saturated boundary condition, elements will span a region from initial to full saturation early in the transient. A conservative estimate of the minimum time increment is found by choosing the initial saturation of 0.05. From this, we compute $k_s$, $ds/du$, and a value of $\mathrm{\Delta }t$ of about 70 sec. We find, in practice, that an initial increment of 50 sec is adequate to avoid oscillations in this problem. For the remaining input files the initial time increment is chosen as discussed in Partially saturated flow in a porous medium since we have the same material properties and spatial discretization.

In this analysis the prevailing pore pressure in the medium approaches the magnitude of the stiffness of the material skeleton elastic modulus. When reduced-integration elements are used in such cases, the default choice for the hourglass stiffness control, which is based on a scaling of skeleton material constitutive parameters, may not be adequate to control hourglassing in the presence of the relatively large pore pressure fields. An appropriate hourglass control setting in these cases should scale with the expected magnitude of pore pressure changes over an element and must be defined explicitly by the user.

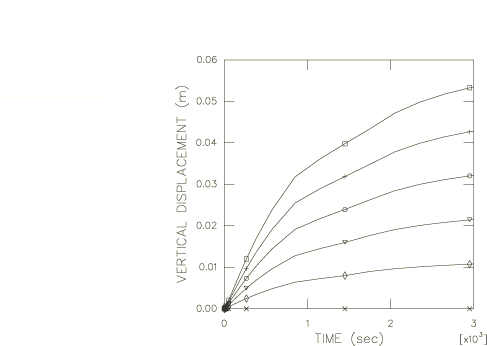
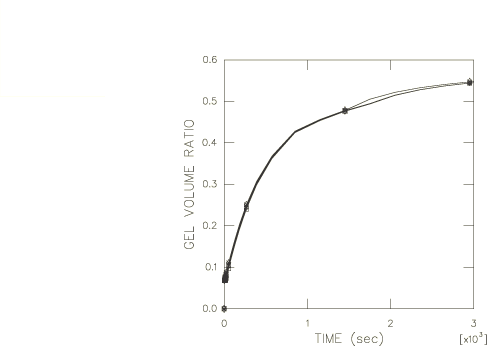
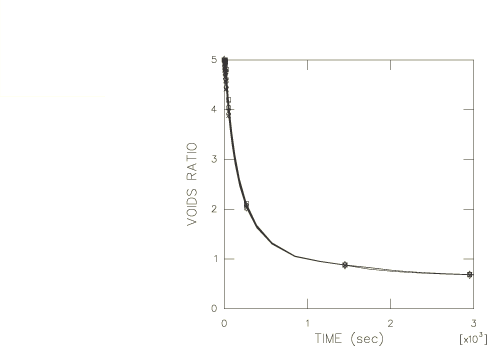
Geometric nonlinearities are considered in the analysis since we expect large deformations due to the growth of the gel particles.