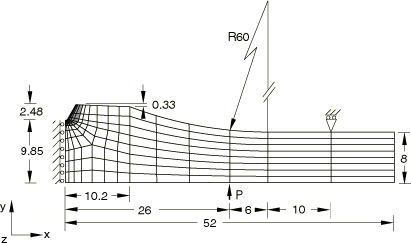
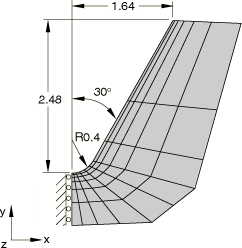
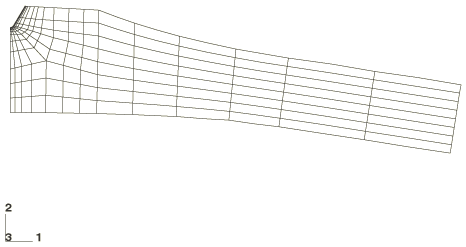
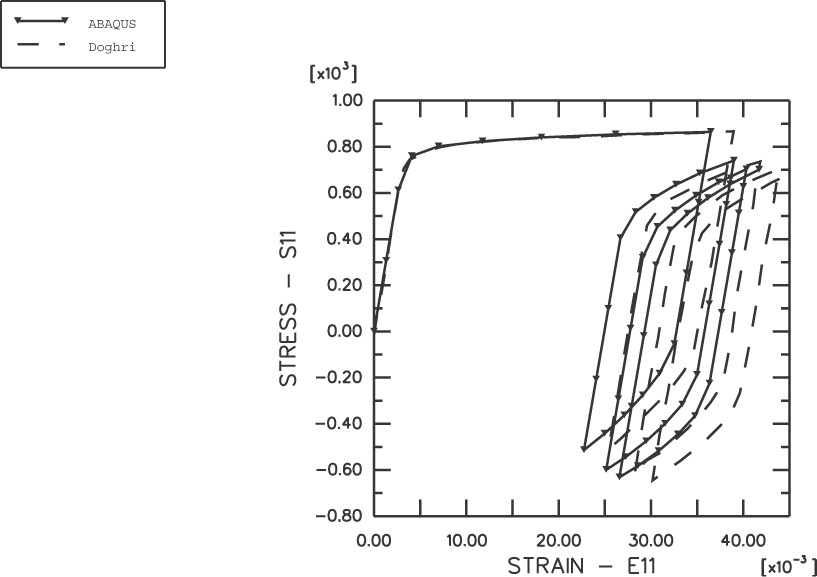
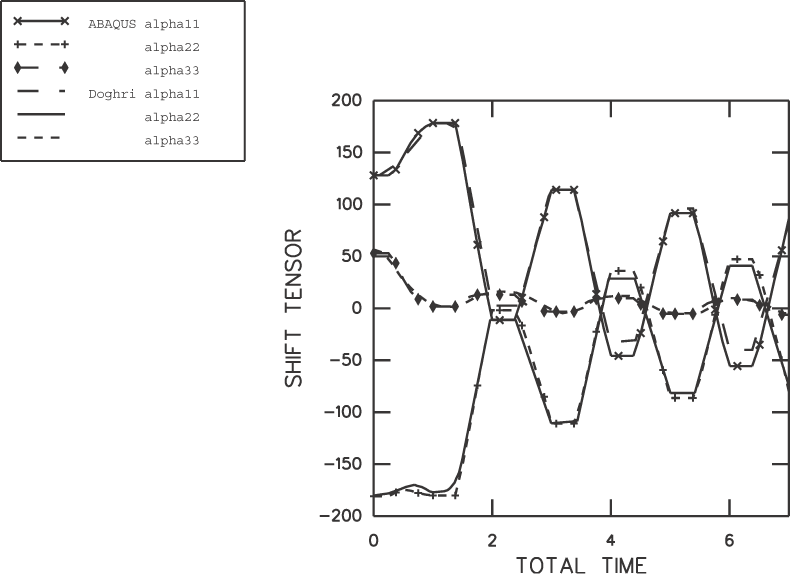
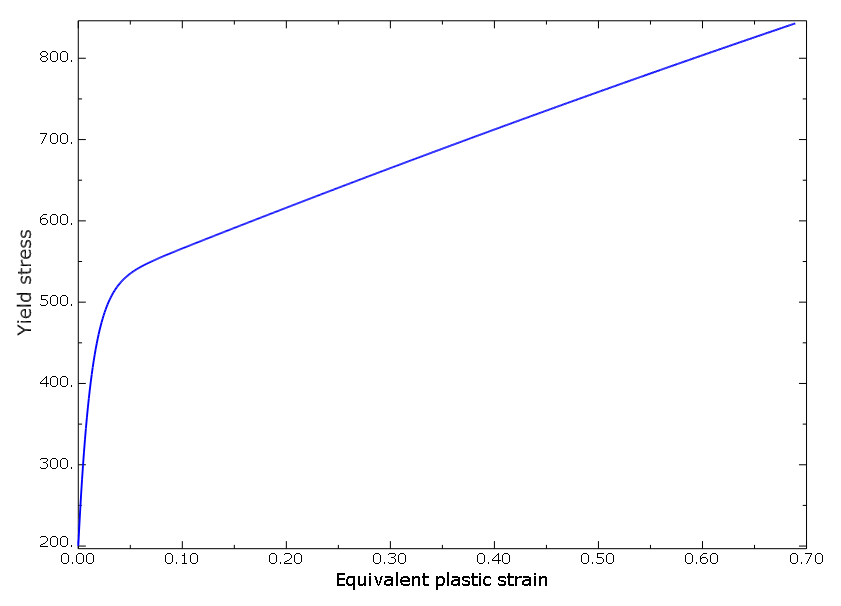
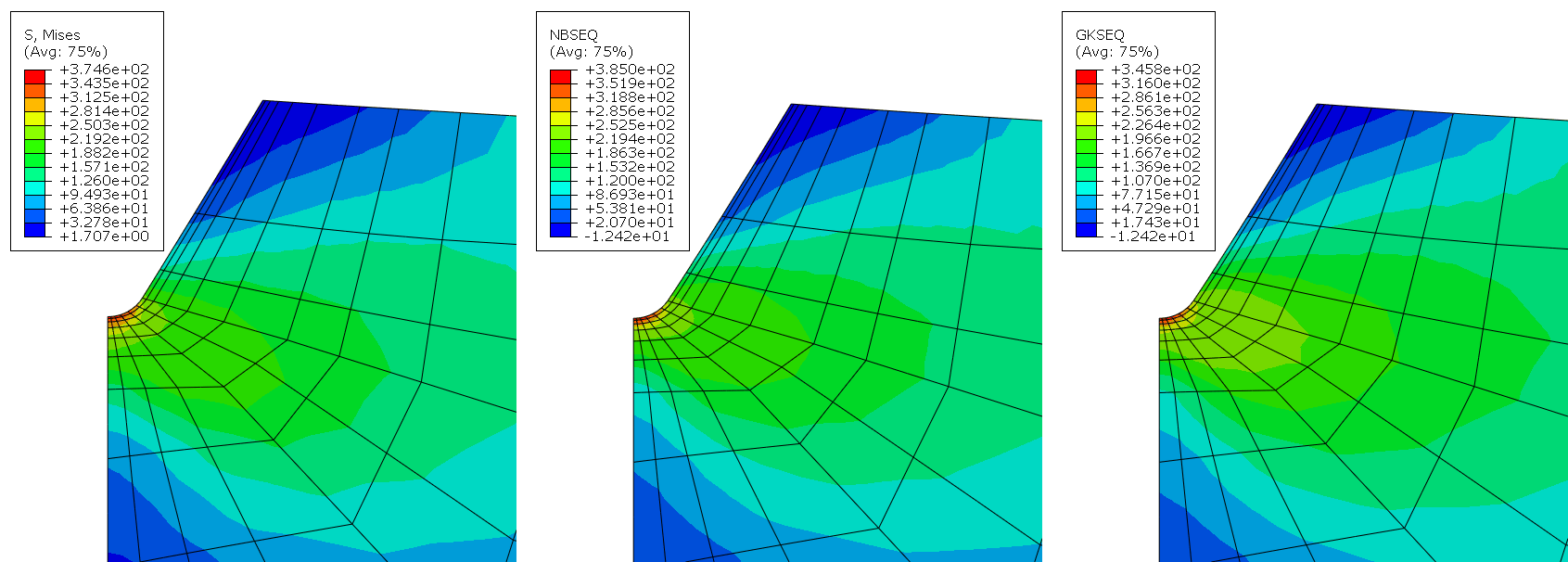
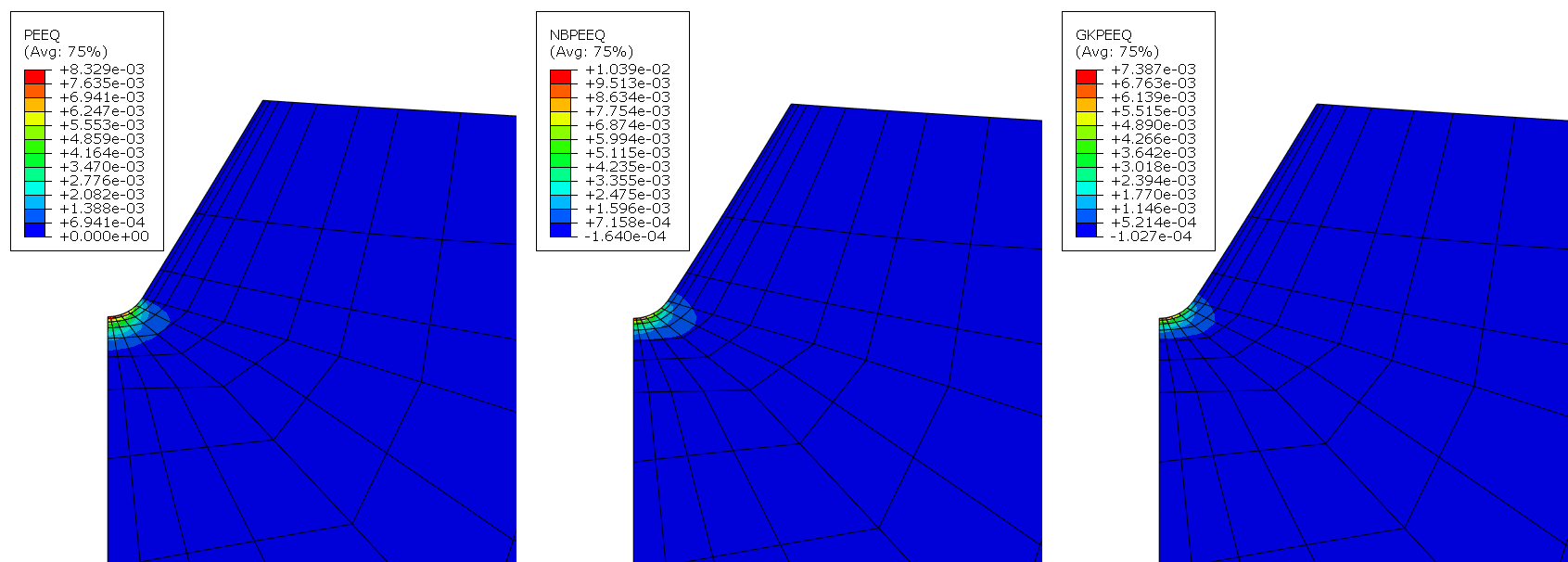
The material properties reported by Doghri (1993) for a low-carbon (AISI 1010), rolled steel are used in this example.
A Young's modulus of E= 210 GPa and a Poisson's ratio of = 0.3 define the elastic response of the material. The initial yield stress is = 200 MPa.
The nonlinear evolution of the center of the yield surface is defined by the equation
where is the backstress, is the size of the yield surface (size of the elastic range), is the equivalent plastic strain, and C = 25.5 GPa and = 81 are the material parameters that define the initial hardening modulus and the rate at which the hardening modulus decreases with increasing plastic strain, respectively. The quantity 257 MPa defines the limiting value of the equivalent backstress ; further hardening is possible only through the change in the size of the yield surface (isotropic hardening).
The isotropic hardening behavior of this material is modeled with the exponential law
where is the size of the yield surface (size of the elastic range), = 2000 MPa is the maximum increase in the elastic range, and b = 0.26 defines the rate at which the maximum size is reached as plastic straining develops.
The material used for this simulation is cold rolled. This work hardened state is represented by specifying an initial equivalent plastic strain = 0.43 (so that = 411 MPa) and an initial backstress tensor