Problem description
The physical problem considered here is that of a cantilever beam subjected to thermal and mechanical loading with simple hydrogen concentration boundary conditions. Diffusion is driven by the gradients of temperature and equivalent pressure stress. In this example we are concerned with the hydrogen diffusion aspect of the problem; fictitious diffusion properties are chosen.
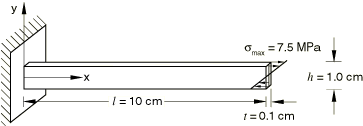
The problem geometry and boundary conditions are shown in Figure 1, and the finite element mesh is shown in Figure 2. The specimen is 1-mm thick, 10-mm high, and 100-mm long. The hydrogen concentration is specified at both ends of the beam at the neutral axis, and flux through all other surfaces is assumed to be zero.
The sequentially coupled mass diffusion analysis consists of a coupled temperature-displacement analysis followed by a mass diffusion analysis. Equivalent pressure stresses from the temperature-displacement analysis are written to the results file as nodal averaged values. Temperatures from the temperature-displacement analysis are stored on the results file as nodal values. Subsequently, these pressure stress and temperature fields are read in during the course of the mass diffusion analysis to provide driving mechanisms for mass diffusion.
The material properties for mass diffusion were selected to verify the sequentially coupled mass diffusion procedure and are not intended to model true properties of the material. Solubility, s, is defined as unity so that concentration, c, and normalized concentration, , are equivalent. This assumption is acceptable since no material interfaces are present. Diffusivity, D, is specified as 3.6 × 10−6m2/h; temperature dependence of the diffusivity is not accounted for in this example. Stress-assisted diffusion is specified by defining the pressure stress factor, , as
where is the normalized concentration. The Soret effect factor, , which allows temperature-driven mass diffusion, is defined as
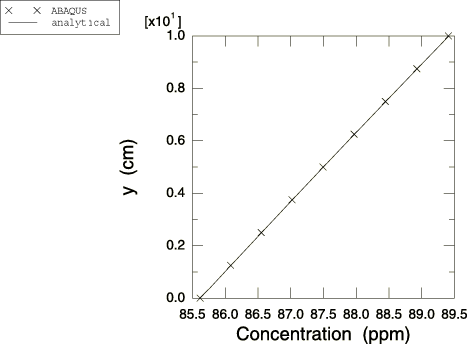
where () is the absolute temperature, is the maximum gradient of equivalent pressure stress applied to the beam, and is the maximum temperature gradient applied to the beam. This definition of , although not realistic, allows the mass diffusion behavior to be verified easily when both equivalent pressure stress and temperature are read from the results file: when the gradients of both temperature and equivalent pressure stress are applied simultaneously, these properties indicate that mass diffusion should be driven by concentration gradients alone. The concentration dependence of and is entered in Abaqus in tabulated form, as shown in the input listings.
The following properties are also used in the coupled temperature-displacement analysis: elastic modulus, 2.0 × 1011Nm−2; Poisson's ratio, 0.3; and conductivity, 1.0 × 103W m−1K−1.
The specimen is initially at a constant temperature of 273 K, and an initial concentration of 50 ppm is applied over the entire beam. In the first step the concentration at the free end of the beam is ramped to 100 ppm over the step and the steady-state distribution is determined. During the second step a bending moment is applied at the end of the beam to achieve a maximum tensile stress of 7.5 MPa, corresponding to a maximum equivalent pressure stress gradient of 650 MPa/m. The bending moment is applied as a ramp over 10% of the mass diffusion step and maintained until steady-state mass diffusion conditions are reached. In the third step the temperature field is applied to the mass diffusion analysis. A temperature gradient of 3.0 × 104 K/m is applied as a ramp over 10% of the mass diffusion step and maintained until steady-state mass diffusion conditions are reached.