Problem description
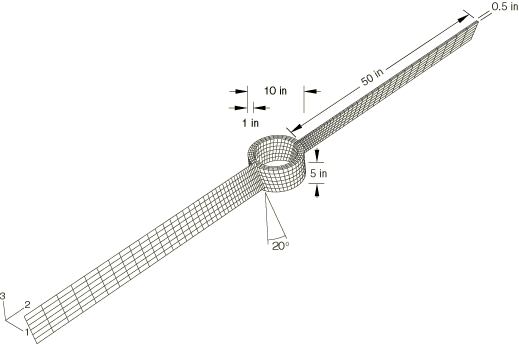
The rotation of a propeller that has an initial spin rate of 314.16 rad/s (3000 rpm or 50 revolutions per second) is simulated for a duration of 2 seconds. The propeller consists of a rigid central hub and two deformable blades, as shown in Figure 1. The hollow cylindrical hub is 5 inches (0.1270 m) long and has inner and outer radii of 4 inches (0.1016 m) and 5 inches (0.1270 m), respectively. The blades are 0.5 inches (0.0127 m) thick and span 50 inches (1.27 m). The blades make a 20° angle with the central axis of the propeller. The entire propeller assembly is made of steel with the following properties:
| Young's modulus = 30.0 × 106 psi (207 GPa) |
| Poisson's ratio = 0.3 |
| Density = 7.3 × 10−4 lbf-sec2/in4 (7800 kg/m3) |
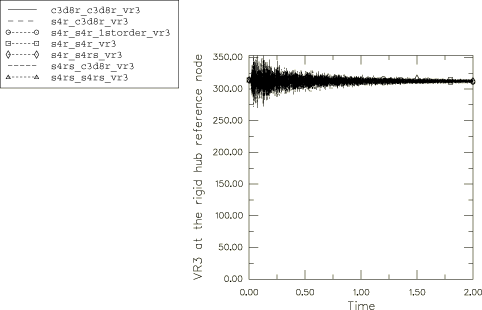
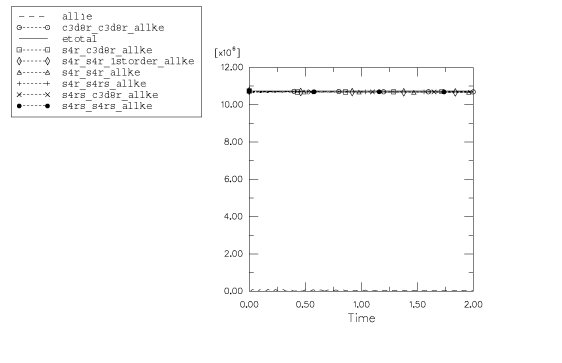
The propeller is assumed to be spinning in a vacuum with zero initial stresses. Once the stress state due to the centrifugal loading is established, the solution (displacements, stresses, and strains) is expected to oscillate about this state with time. The mass moment of inertia of the propeller about its central axis increases slightly due to the stretching of the blades in the mean stress state under the centrifugal loading. Since the angular momentum is conserved, the increase in the mass moment of inertia is expected to result in a mean spin rate that is slightly less than the initial spin rate.
Meshing of the blades and the hub
In this study the hub region of the propeller is modeled as a rigid body that is discretized with C3D8R elements. The propeller blades are discretized with either S4R, S4RS, or C3D8R elements. Each propeller blade is discretized with a single element type, although two different element types can be used for the two blades. This leads to six different model permutations, all of which are studied in this example.
Order of accuracy in element formulation
Since the finite elements undergo a large number (> 5) of rigid body revolutions, the second-order accurate element formulation is specified in the section controls. The six models of the propeller problem with different element combinations are analyzed using second-order accurate elements. In addition, the model with both blades discretized using S4R elements is also analyzed using the default first-order accurate element formulation option.