Minimizing the Mass of the Upright Example (MIMP)

This example shows the usage of the MIMP material interpolation.

See Also
About Checking the Quality of the Optimization Result
In Other Guides
Material Interpolation
  1. Define input data and Design Variables: 
    FEM_INPUT
  ID_NAME  = INPUT_FILE
  FILE     = upright_mimp.inp
END_

DV_TOPO
  ID_NAME  = globalDesignArea
  EL_GROUP = "Design_Space1"
END_
  2. Define additional groups by combining (LIST_GROUP) and subtracting (LIST_SUBTRACT_GROUP) existing groups: 
    GROUP_DEF
  ID_NAME = globalFrozenRegion
  TYPE    = ELEM
  FORMAT  = LIST_GROUP
  LIST_BEGIN
"Functional Region1",
"Functional Region2",
"Functional Region3",
"Functional Region4",
"Functional Region5",
"Functional Region7",
"Functional Region8"
END_

GROUP_DEF
  ID_NAME = designAreaNonFrozen
  TYPE    = ELEM
  FORMAT  = LIST_SUBTRACT_GROUP
  LIST_BEGIN
"Design_Space1", globalFrozenRegion
END_
  3. To use mass in the objective, define a Design Response (DRESP) as follows: 
    DRESP
  ID_NAME    = TASK_MASS
  DEF_TYPE   = SYSTEM
  TYPE       = WEIGHT
  EL_GROUP   = "Design_Space1"
  GROUP_OPER = SUM
END_
  4. Define the Objective Function (OBJ_FUNC) as follows: 
    OBJ_FUNC
  ID_NAME = MY_OBJECTIVE
  DRESP   = TASK_MASS
  TARGET  = MIN
END_
  5. To define the stress, displacement and frequency constraints, do the following:
    1. Define the corresponding design responses (Here, one DRESP each for stress and displacement is shown instead of the total 4): 
      DRESP
  ID_NAME  = StressConstraint1_LC1
  DEF_TYPE = SYSTEM
  TYPE     = SIG_TOPO_MISES
  EL_GROUP = designAreaNonFrozen
  LC_SET   = ALL,1,ALL
END_

DRESP
  ID_NAME  = DisplacementConstraint1_LC1
  DEF_TYPE = SYSTEM
  TYPE     = DISP_ABS
  ND_GROUP = "Displacement Constraint6"
  LC_SET   = ALL,1,ALL
END_

DRESP
  ID_NAME  = eig1
  DEF_TYPE = SYSTEM
  TYPE     = DYN_FREQ
  UPDATE   = EVER
  LC_SET   = MODAL,3,1
END_
  6. Define the respective stress, displacement and frequency constraints (CONSTRAINT) (Here, one CONSTRAINT each for stress and displacement is shown instead of the total 4): 
    CONSTRAINT
  ID_NAME   = CONSTRAINT_StressConstraint1_LC1
  DRESP     = StressConstraint1_LC1
  MAGNITUDE = ABS
  LE_VALUE  = 250000000.0
END_

CONSTRAINT
  ID_NAME   = CONSTRAINT_DisplacementConstraint1_LC1_MAX
  DRESP     = DisplacementConstraint1_LC1
  MAGNITUDE = ABS
  LE_VALUE  = 0.00012
END_

CONSTRAINT
  ID_NAME   = FreqCon
  DRESP     = eig1
  MAGNITUDE = ABS
  GE_VALUE  = 100.
END_
  7. Define frozen regions (Here, one exemplary DVCON is shown): 
    DVCON_TOPO
  ID_NAME    = FunctionalRegion1
  CHECK_TYPE = FROZEN
  EL_GROUP   = globalFrozenRegion
END_
  8. Reference the Objective Function, Design Variables and Constraints as well as DVCONs in the OPTIMIZE command: 
    OPTIMIZE
  ID_NAME    = OptimizationTask
  STRATEGY   = TOPO_SENSITIVITY
  DV         = globalDesignArea
  OBJ_FUNC   = OBJECTIVE_MIN_MASS
  CONSTRAINT = CONSTRAINT_StressConstraint1_LC1
  CONSTRAINT = CONSTRAINT_StressConstraint1_LC2
  CONSTRAINT = CONSTRAINT_DisplacementConstraint1_LC1_MAX
  CONSTRAINT = CONSTRAINT_DisplacementConstraint1_LC2_MAX
  CONSTRAINT = FreqCon
  DVCON      = FunctionalRegion
END_
  9. Deactivate the function to automatically freeze specific regions and set the minimum initial density to 0.1. In addition set the material interpolation to MIMP: 
    OPT_PARAM
  ID_NAME             = OptimizationTask_PARAMS
  OPTIMIZE            = OptimizationTask
  AUTO_FROZEN         = OFF
  MIN_INITIAL_DENSITY = 0.1
  MAT_INTERPOLATION   = MIMP
END_

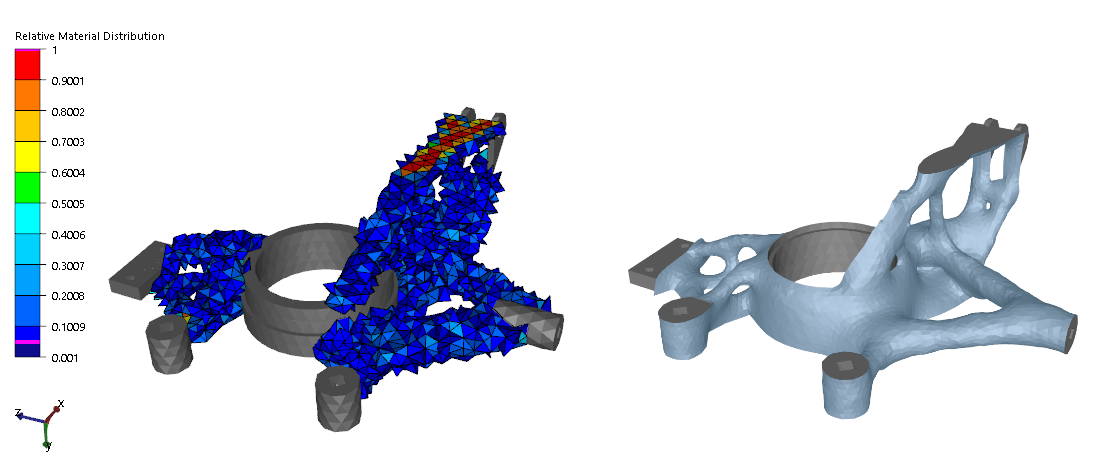
The optimization result looks as follows:

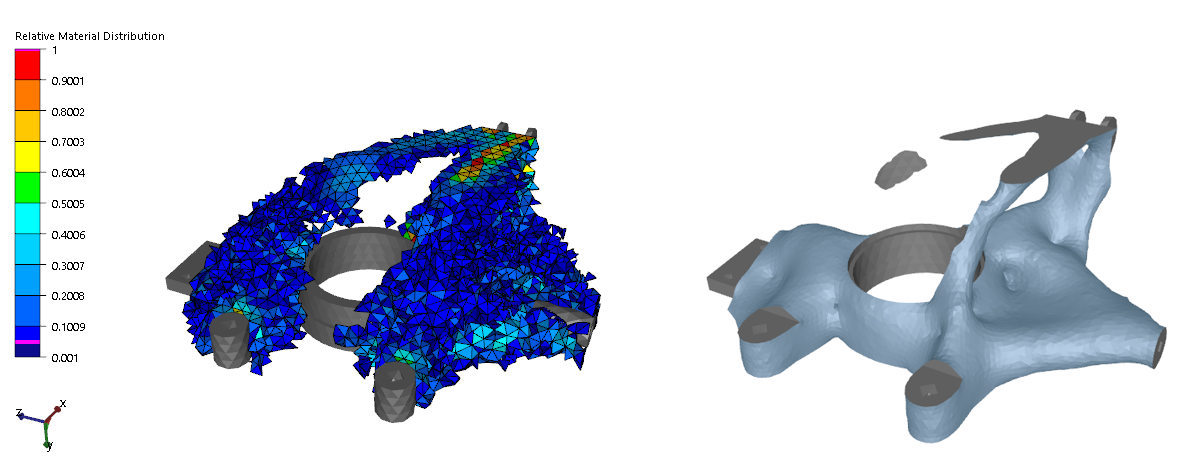
The result using the default interpolation can be seen below. The structure does not look converged and too much intermediate density is present. Also, the smoothed result is not valid:



Valid result using the MIMP interpolation. A layer of low density elements is present, but as in the smoothed image, the structure is well connected and converged: