Normal Distribution

The Normal or Gaussian distribution is a two-parameter distribution defined in terms of the mean $μ$ and standard deviation $σ$ of the random variable $X$ .

The normal distribution probability density function is

f_{X} (x) = \frac{1}{σ \sqrt{2 π}} \exp [\frac{- {(x - μ)}^{2}}{2 σ^{2}}] - \infty < x < \infty .

The distribution function corresponding to the density function of the previous equation is given by

F_{X} (x) = \int_{- \infty}^{x} \frac{1}{σ \sqrt{2 π}} \exp [\frac{- {(t - μ)}^{2}}{2 σ^{2}}] d t = Φ (\frac{x - μ}{σ})

where 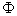is the standard normal distribution function ( $μ = 0$ and $σ = 1$ ) defined by

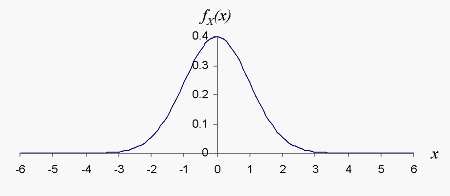
Φ (x) = \int_{- \infty}^{x} \frac{1}{σ \sqrt{2 π}} \exp (\frac{- t^{2}}{2}) d t

The corresponding standard normal density function, illustrated in the following figure, is given by

φ (x) = \frac{1}{σ \sqrt{2 π}} \exp (- \frac{x^{2}}{2}) .

The normal distribution, which is shown in the following figure, is the common “bell curve” distribution, often used for physical measurements, product dimensions, and average temperatures.